John Stone Stone was probably the best known U.S. mathematician during radio's early development. In contrast, Lee DeForest took a much more empirical approach, and had a reputation more for trail-and-error efforts than careful analysis. Both approaches had their strengths and their drawbacks. George H. Clark was a employee of Stone's short-lived radio company, and looking back on those days, noted in a biography he wrote about Stone that the company had something of an excess of theoreticians, and might have benefited by having more practical experience. Meanwhile, Frank Butler, writing about his work as an assistant to DeForest at the Saint Louis Exposition in 1904, reported that "Mathematics had no place in the embryo radio of those days", which helps explain DeForest's reputation for producing somewhat crude equipment. In the end, radio's future's would be dependant on a mixture of the theoretical and practical experience.
At the 1904 Saint Louis Exposition, Stone presented a paper outlining some mathematical foundations of radio transmissions. It is interesting to note that at one point Stone stated that as radio signals spread out, their strength diminished with the square of their distance. This was actually an error, as groundwave radio signal strength actually tended to diminish directly with distance, as they spread across the earth in just two dimensions. With his extensive practical experience, DeForest noticed that Stone's statement was at variance with his work -- this would have been especially noticeable where the signals traveled over highly conductive paths, such as sea water. However, because he had never made careful measurements, DeForest was unable to say for certain exactly how quickly the signal strengths weakened.
Transactions of the 1904 Saint Louis International Electrical Congress, Volume III, pages 556-558:
THE THEORY OF WIRELESS TELEGRAPHY.
______
BY JOHN STONE STONE.
______
 This theory regards the vertical transmitting oscillator of wireless telegraphy as one-half of a Hertz oscillator normal to the earth's surface, which must be regarded as practically infinitely conductive in the immediate neighborhood of the oscillator, or for about a quarter of a wave length from the point at which the oscillator is connected to the surface of the earth. By this theory, therefore, the waves of wireless telegraphy are developed in exactly the same manner as if the vertical oscillator and its electrical image below the surface of the earth together formed the real oscillator of which the surface of the earth is the equatorial plane.
This theory regards the vertical transmitting oscillator of wireless telegraphy as one-half of a Hertz oscillator normal to the earth's surface, which must be regarded as practically infinitely conductive in the immediate neighborhood of the oscillator, or for about a quarter of a wave length from the point at which the oscillator is connected to the surface of the earth. By this theory, therefore, the waves of wireless telegraphy are developed in exactly the same manner as if the vertical oscillator and its electrical image below the surface of the earth together formed the real oscillator of which the surface of the earth is the equatorial plane.
A graphical representation of this theory is given in Figs. 1 and 2.
This theory which for convenience may be termed the "Electrical Image" theory, bears a close resemblance to that mode of treating a single wire or grounded telegraph or telephone circuit as one-half of a two-wire or metallic circuit which was first suggested by Mr. Oliver Heaviside.2 He conceives a metallic circuit such as that shown in Fig. 3, cut in half longitudinally by an infinitely conducting plane at zero potential as shown in Fig. 4. Since the points on the metallic circuit cut by the plane would normally be at zero potential, no change in the distribution of currents results from the connection with the infinitely conductive plane. A little consideration will also show that the electrostatic capacity and inductance of the circuit will moreover remain unchanged. The surface of the earth is not infinitely conductive, however, and therefore neither the assumptions made in the electrical image theory of the transmitting oscillator of wireless telegraphy nor the electrical image theory of the grounded telephone line are completely justified,3 though the conditions of the theory may be more nearly approximated in the case of wireless telegraphy, as will become apparent later.
Before proceeding to a consideration of a more comprehensive theory, some of the more obvious conclusions to be drawn from this theory may well be stated. These are:
1). The waves which emanate from the vertical oscillator are horizontally polarized electromagnetic waves.
2). The energy of these waves will diminish as the square of the distance from the oscillator if the surface of the earth be assumed to be flat.
pages 576-577:
DISCUSSION.
Dr. LEE DE FOREST: I wish to indorse the remarks of the last speaker on the value and painstaking care represented by this work of Mr. Stone. Perhaps the most interesting feature that he brought out was the question of the quantitative dissipation of energy from the source relative to the distance. As applied practically over distances varying from a quarter of a mile to three hundred miles, over land and over sea, I have found no indication whatever that the received energy falls off as the inverse square. In fact, I cannot reconcile that theory with experience. Of course, you all appreciate the practical impossibility of making strictly quantitative measurements from the source of a two-horse power station, where the energy received on a single wire is practically infinitesimal. The nearest we can approximate to it is by the telephone and the human ear trained to make comparisons. The human ear is not a strictly quantitative instrument by any means, and the subjective impression made varies inversely as the energy received in the telephones. I cannot reconcile the theory with practical experiments. I would like to hear from any other gentlemen present if their experience has been in line with my own or to the contrary.
Mr. JOHN HESKETH: In reference to the remark made as to the apparent discrepancy between what was assumed to be the theoretically correct values of the energy received in the antennæ and the actual results obtained when using a telephone receiver and the ear for quantitative measurements, I do not doubt that Dr. De Forest will grant that the telephone receiver and the ear in conjunction are most unreliable as quantitative measuring instruments. Results obtained by those means cannot be taken as either confirming or contradicting theory, unless the telephone received sound is very, very widely different in two cases under comparison. I believe that recently a quantitative method has been tried by Mr Duddell, of oscillograph fame, and that results which are strictly quantitative will shortly be published.
2. Heaviside, "Electrical Papers," II, Art. 41, App. A, pp. 323 to 334. Also see footnote to Ab. IX, p. 140, Vol. 1.
3. Heaviside, Vol. II, pp. 220 to 221. Vol. II, pp. 302 to 307.
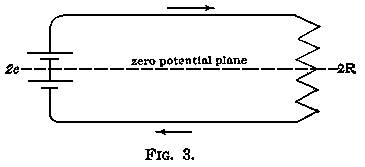
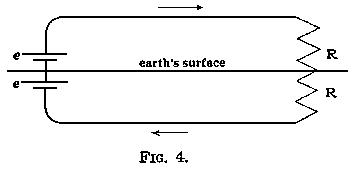
 This theory regards the vertical transmitting oscillator of wireless telegraphy as one-half of a Hertz oscillator normal to the earth's surface, which must be regarded as practically infinitely conductive in the immediate neighborhood of the oscillator, or for about a quarter of a wave length from the point at which the oscillator is connected to the surface of the earth. By this theory, therefore, the waves of wireless telegraphy are developed in exactly the same manner as if the vertical oscillator and its electrical image below the surface of the earth together formed the real oscillator of which the surface of the earth is the equatorial plane.
This theory regards the vertical transmitting oscillator of wireless telegraphy as one-half of a Hertz oscillator normal to the earth's surface, which must be regarded as practically infinitely conductive in the immediate neighborhood of the oscillator, or for about a quarter of a wave length from the point at which the oscillator is connected to the surface of the earth. By this theory, therefore, the waves of wireless telegraphy are developed in exactly the same manner as if the vertical oscillator and its electrical image below the surface of the earth together formed the real oscillator of which the surface of the earth is the equatorial plane.

